Drying Characteristics and Shrinkage Model Analysis of Pitaya Heat Pump Drying
-
摘要: 为了优化火龙果热泵干燥工艺及提升干燥后产品的品质，本文研究了干燥温度、切片厚度和相对湿度对火龙果热泵干燥特性和体积比的影响，并确定了最佳收缩动力学模型，从而可以预测火龙果在不同热泵干燥条件下的体积变化规律。结果表明，干燥温度越高、切片厚度和相对湿度越小，干燥速率越大；其中，干燥温度对干燥速率影响最大，切片厚度影响最小；体积比随干燥温度的升高、切片厚度和相对湿度的减小而减小；对比分析5种薄层干燥模型，Quadratic模型可以精确描述火龙果热泵干燥过程中的体积收缩规律，计算值相对于试验值的平均误差为5.01%；在本文所述热泵干燥条件下，通过阿累尼乌斯方程计算出火龙果的收缩活化能为27.185 kJ/mol。本研究借助体积收缩模型优化热泵干燥工艺参数并获得更合适体积的干制品，可为火龙果在热泵干燥过程中体积收缩规律提供技术支持。Abstract: In order to optimize the pitaya heat pump drying process and improve the quality of dried products, the effects of drying temperature, slice thickness and relative humidity on heat pump drying characteristics and the volume ratio of pitaya were studied. The optimal shrinkage dynamic model was determined to predict the volume variation law under different heat pump drying conditions. The results demonstrated that the drying rate increased with the increase of drying temperature, the decrease of slice thickness and relative humidity. The drying temperature had the greatest effect on it while the slice thickness had the least. The volume ratio decreased with the increase of drying temperature and the decrease of slice thickness and relative humidity. Comparing and analyzing the five thin-layer drying models, the Quadratic model was determined as the most accurate to describe the volume ratio law in the pitaya heat pump drying process. The average error of the calculated value was 5.01% compared with the test value. Under the heat pump drying conditions described in this paper, the contraction activation energy of the pitaya was calculated to be 27.185 kJ/mol by Arrhenius equation. Based on the volume shrinkage model, the process parameters of heat pump drying could be optimized and the dry products with more appropriate volume could be obtained. This study could provide technical support for the volume shrinkage law of pitaya in the heat pump drying process.
-
Keywords:
- pitaya /
- heat pump drying /
- drying characteristics /
- shrinkage dynamics /
- activation energy
-
火龙果营养价值高，口味独特，是世界上最受欢迎的水果之一[1-2]。随着火龙果种植规模的扩大，火龙果产量逐年增加。但是，火龙果含水量高，非常容易腐烂，火龙果集中采摘后往往会因销售不佳而导致果实腐烂变质，造成一定的资源浪费。干燥是火龙果的主要加工方式之一，干燥后的产品可以延长贮藏时间并维持产品质量，以提高火龙果利用率[3]。热泵干燥温度较低，干燥过程中水分蒸发速率较低，能较好地维持干制品原有的营养成分、色泽及形态，因此被认为是最合适火龙果干燥的方法[4-5]。
在不同干燥条件下干制品的体积比是影响其品质的重要指标，通过研究收缩动力学模型可以分析热泵干燥过程中样品的体积变化规律，基于此规律可以获得最优体积的干制品并优化热泵干燥工艺参数。目前，已有学者对果蔬的收缩动力学模型及热泵干燥特性进行了深入研究。Mayor等[6]研究了不同食品在对流干燥过程中的收缩现象，对收缩机理进行了物理描述，并通过对数据的回归分析获得经验模型；刘鹤等[7]发现Weibull函数可以准确描述马铃薯的降速干燥过程，在给定试验条件下的收缩活化能为46.44 kJ/mol；陈良元等[8]通过比较不同种类的薄层干燥模型发现，Hatamipour和Quadratic模型都可以较好描述茄子在干燥过程中的体积收缩特性；Dhalsamant等[9]发现是否考虑收缩变形对马铃薯热风干燥过程的模拟结果有很大影响，考虑收缩可以有效提升热空气与物料表面对流换热系数的计算精度；Burmester等[10]通过对咖啡豆等材料的研究发现，引入收缩动力学模型可以更好地预测农产品干燥过程中体积收缩的规律；Sandoval等[11]对比分析了不同收缩动力学模型，深入研究了马铃薯热风干燥过程中的脱水过程，并对产品品质进行了优化。
目前基于热泵干燥方法研究火龙果干燥特性和收缩动力学模型的文献较少，该研究对优化火龙果热泵干燥工艺及获取最佳体积的干制品有重要意义。本文研究了不同干燥温度、切片厚度和相对湿度对火龙果热泵干燥特性和体积比的影响；分析了火龙果在热泵干燥过程中水分含量和体积的变化规律，得出最佳收缩动力学模型，并验证模型的准确性；基于菲克定律，通过阿累尼乌斯方程计算火龙果的收缩活化能；以期为提高产品品质及优化热泵干燥工艺参数提供技术支持。
1. 材料与方法
1.1 材料与仪器
白心火龙果 采购于郑州丹尼斯超市，满足大小适中，体积相似，无病虫害和机械损伤的条件，购买后放入2~4 ℃冷藏室内备用。
CG-05HA空气能热泵箱式一体节能烘干机 广东创陆制冷科技有限公司；FBS-750A快速水分仪 厦门弗布斯检测设备有限公司；139型水果切片机 广州恒纳奶茶餐饮设备；ES500精密电子天平 天津市德安特传感技术有限公司；91514型数显型游标卡尺 上海世达工具有限公司。
1.2 实验方法
将火龙果清洗后去皮，切成边长为50 mm，厚度分别为8、9、10 mm左右的薄片；取四片为一组，擦去表面水分后称重，并使用快速水分仪测量火龙果鲜果水分含量；将热泵干燥机预热30 min后把每组火龙果样品放入干燥。总共进行了9组实验，设定切片厚度为9 mm，相对湿度为20%；干燥温度60 ℃，相对湿度为20%和干燥温度60 ℃，切片厚度9 mm，比较不同干燥温度、切片厚度及相对湿度对火龙果热泵干燥特性及体积收缩的影响。每隔1 h测量一次克重和体积，干燥至每组样品重量不再变化时，干燥结束；计算干燥成品的干燥特性和体积比；对体积比进行拟合得出可以准确描述干制品体积收缩规律的体积收缩模型，并计算活化能。
1.3 评价指标的测定
1.3.1 干基含水率
干基含水率计算公式如式（1）[12]。
$$\rm {W_d}{\text{ = }}\frac{{{m_w}{{ - }}{m_d}}}{{{m_d}}} $$ (1) 式中：Wd为t时刻的干基含水率；mw为t时刻湿火龙果质量；md为火龙果的绝干质量。
1.3.2 干燥速率
干燥速率的计算公式如式（2）[13]。
$$\rm {D_R}{\text{ = }}\frac{{{M_t}{{ - }}{M_{t+dt}}}}{{{d_t}}} $$ (2) 式中：DR为干燥速率，g/h；Mt+dt和Mt分别为样品在t+dt和t时刻的干基含水率。
1.3.3 水分比
水分比的计算公式如式（3）[14]。
$$\rm MR{\text{ = }}\frac{{{M_t}{{ - }}{M_e}}}{{{M_0}{{ - }}{M_e}}} $$ (3) 式中：MR为水分比；Mt为干燥过程中t时刻样品的干基含水率；Me和M0分别为样品的平衡干基含水率和初始干基含水率，Me相当于Mt和M0来说非常小，可忽略不计，水分比可以根据式（4）[15]计算。
$$\rm MR{\text{ = }}\frac{{{M_t}}}{{{M_0}}} $$ (4) 1.3.4 体积比
因火龙果片干燥后形变较小，干燥前后的形状较为规则，故将待测样品近似为长方体，用长方体体积计算公式计算体积。体积比的计算公式如式（5）[16]。
$$\rm {{\text{V}}_{\text{R}}} = \frac{{{{\text{V}}_{\text{t}}}}}{{{{\text{V}}_{R0}}}} $$ (5) 式中：VR为体积比；Vt和VR0分别为干燥过程中t时刻和初始时刻样品的体积。
1.3.5 体积收缩模型
火龙果片的干燥属于薄层干燥，为了得到最符合火龙果热泵干燥中体积变化规律的体积收缩模型，本文选取5种常用的体积收缩模型对其拟合分析[7-8,17-18]，进行量化评价后，得到适应度最佳的体积收缩模型，如表1所示。
表 1 体积收缩模型Table 1. Volume shrinkage model模型序号 模型名称 模型方程 1 Exponential $\rm {V_R} = aexp\left( {bMR} \right)$ 2 Quadratic $\rm {V_R} = a+bMR{\text{ } }+cM{R^2}$ 3 Hatamipour $\rm {V_R} = a+b{\text{MR} }$ 4 1阶 $\rm {V_R} = {V_{R0} }exp\left( { - kt} \right)$ 5 Weibull分布函数 $\rm { {\text{V} }_{\text{R} } } = exp[ - {\left(\dfrac{t}{\alpha }\right)^\beta }]$ 为了评价模型的精确度，本文采用决定系数R2、残差平方和RSS和卡方χ2这三个常用的指标进行评价与最优模型的选择。评价参数的计算公式[19-20]如下所示。
$$ {R^2} =\rm 1 - \frac{{\displaystyle\sum\limits_{i = 1}^n {{{({{\text{V}}_{Rcal,i}} - {V_{Rexp,i}})}^2}} }}{{\displaystyle\sum\limits_{i = 1}^n {{{(\overline {{V_{{\text{Rcal,}}i}}} - {V_{Rexp,i}})}^2}} }} $$ (6) $$\rm RSS = \sum\limits_{i = 1}^n {{{({V_{Rcal,i}} - {V_{Rexp,i}})}^2}} $$ (7) $$\rm {\chi ^2} = \frac{{\displaystyle\sum\limits_{i = 1}^n {{{({{\text{V}}_{\text{R}}}_{cal,i} - {{\text{V}}_{\text{R}}}_{exp,i})}^2}} }}{{n - m}} $$ (8) 式中：VRcal,i为体积比计算值；VRexp,i为体积比试验值；n为试验值个数；m为计算值个数。
1.3.6 收缩活化能
收缩速率常数k的计算公式如式（9）所示。不同干燥温度下火龙果的收缩活化能与收缩速率常数k有关，两者关系如式（10）[21]所示：
$$\rm {\text{k}} = \frac{{{L^2}}}{\alpha } $$ (9) $$\rm \ln k = \frac{{{E_a}}}{R}\left(\frac{1}{{{T_r}+273.15}} - \frac{1}{{T+273.15}}\right)+\ln {k_r} $$ (10) 式中：
$ \alpha $ 为Weibull分布函数中的尺度参数min；Tr和T分别为热泵干燥箱中的额定温度和实际温度，℃；kr为额定速率常数，min−1；L为切片厚度，m。1.4 数据处理
本文中每组试验平行重复3次，试验数据取平均值。采用Excel 2019进行试验数据分析处理，采用Origin 2017进行图片绘制。
2. 结果与分析
2.1 火龙果的热泵干燥特性
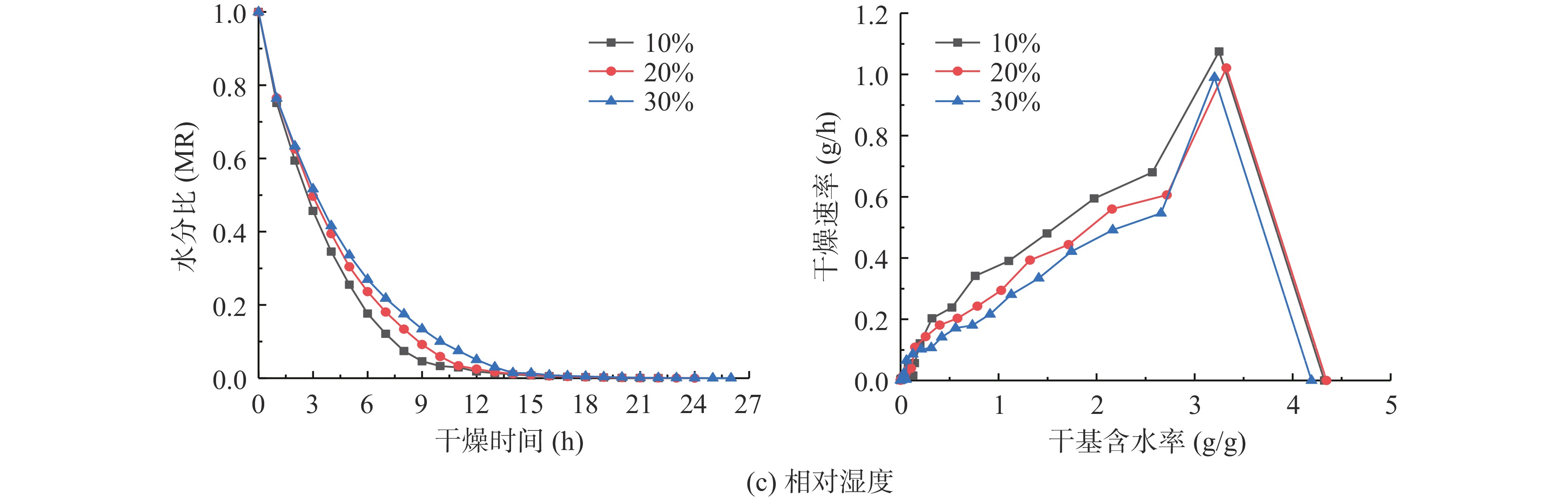
不同干燥条件对火龙果热泵干燥特性的影响见图1。由图1a可知，在切片厚度为9 mm，相对湿度为20%，干燥温度分别为50、60、70 ℃的条件下，火龙果达到最低含水率所需时间分别为28、24、20 h，干燥温度为70 ℃时比其为50 ℃时干燥时间缩短28.57%；由图1b可知，在干燥温度为60 ℃，相对湿度为20%，切片厚度分别为8、9、10 mm的条件下，火龙果达到最低含水率所需时间分别为22、24、25 h，当切片厚度为8 mm时，干燥时间比10 mm缩短了12%；由图1c知，在干燥温度为60 ℃，切片厚度为9 mm，相对湿度分别为10%、20%、30%的条件下，火龙果达到最低含水率所需时间分别为22、24、26 h，相对湿度从10%提高至30%，干燥时间延长了18.2%。此结论与Ramirez等[22-23]对苹果热风干燥特性的研究一致。综上可知，干燥速率随着干燥温度的上升，切片厚度和相对湿度的下降而上升，其中干燥温度对干燥速率的影响最明显，而切片厚度对其影响最小。分析原因是较高的干燥温度和较低的相对湿度分别使切片内和切片外的水蒸气压差增大，导致内部的结合水加速外逸，干燥速率提升；切片厚度的减小使得火龙果内结合水逸出距离缩短，从而提高了干燥速率[24-25]。
![]()
图 1 不同干燥条件下火龙果水分比和干燥速率曲线Figure 1. Moisture ratio and drying rate curve of pitaya at different drying conditions2.2 火龙果的体积比
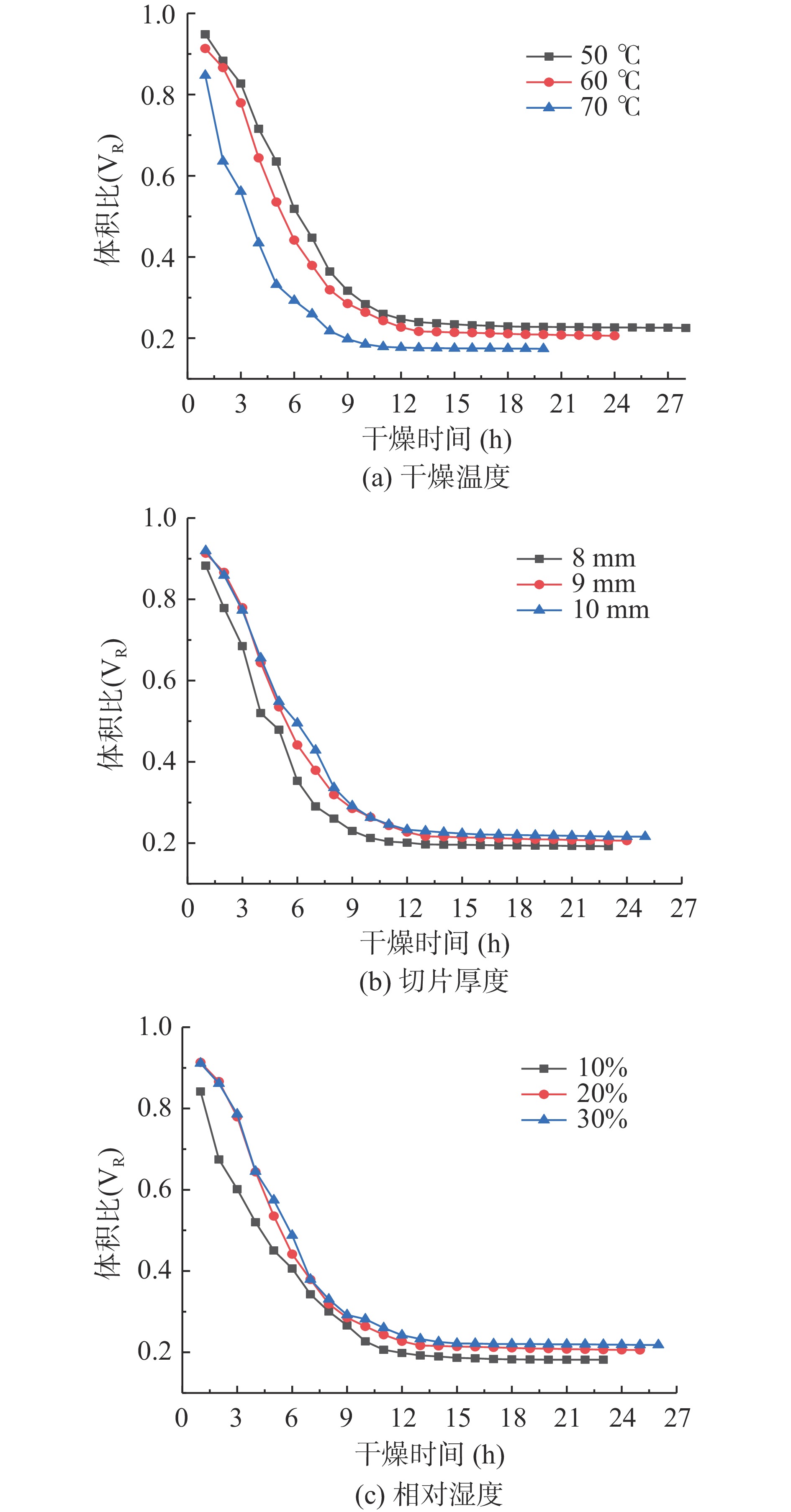
不同干燥条件下火龙果体积比曲线如图2所示。图2a说明在切片厚度和相对湿度恒定时，干燥温度为50、60、70 ℃时体积比分别为22.51%、20.57%和17.37%；图2b表示在干燥温度和相对湿度恒定时，切片厚度为8、9、10 mm时体积比分别为19.2%、20.57%和21.3%；干燥温度和切片厚度固定时，体积比随相对湿度的变化如图2c所示。相对湿度为10%、20%和30%时，体积比分别为18.16%、20.57%和21.81%。分析上述数据可知，火龙果干制品的体积比随干燥温度的上升，切片厚度和相对湿度的下降而下降，且干燥温度对体积比的影响最明显，而切片厚度影响最小。原因是干燥温度的增加，切片厚度和相对湿度的下降增加了干燥强度，加快了水分迁移速率从而增加了火龙果的收缩程度。此结论与徐庚等[18]对芜菁的研究结论一致。
![]()
图 2 不同干燥条件下火龙果体积比曲线Figure 2. Volume ratio curve of pitaya under different drying conditions2.3 火龙果的收缩动力学模型
2.3.1 模型的拟合与最佳模型的选择
将实验数据与5种薄层干燥模型进行拟合，得出火龙果片在不同干燥条件下的模型参数值和评价指标结果如表2所示。
表 2 火龙果片热泵干燥过程体积收缩模型的拟合结果Table 2. Fitting results of shrinkage models of pitaya chips during heat pump drying process序号 模型表达式 模型参数 R2 RSS χ2 Exponential模型 a b 1 $\rm {V_R} = aexp\left( {bMR} \right)$ 0.2538 0.3488 0.9099 0.1256 0.0047 2 0.2447 0.3416 0.9036 0.1148 0.005 3 0.1993 0.3443 0.9535 0.0323 0.0017 4 0.223 0.349 0.9211 0.075 0.0036 5 0.2515 0.3306 0.9044 0.1148 0.0048 6 0.2217 0.3306 0.92 0.0624 0.003 7 0.2463 0.3533 0.9066 0.1121 0.0047 Quadratic模型 a b c 1 $\rm {V_R} = a+bMR{\text{ } }+cM{R^2}$ 0.2081 0.2188 −0.0077 0.9816 0.0256 9.86E-4 2 0.1961 0.2214 −0.0104 0.9871 0.0154 6.99E-4 3 0.1727 0.1549 −9.7E-4 0.9965 0.0024 1.34E-4 4 0.1832 0.197 −0.0067 0.9912 0.0084 4.18E-4 5 0.2038 0.2217 −0.0116 0.9902 0.0118 5.14E-4 6 0.1832 0.195 −0.0108 0.996 0.0031 1.55E-4 7 0.202 0.2099 −0.0063 0.9769 0.0274 0.0011 Hatamipour模型 a b 1 $\rm {V_R} = a+b{\text{MR} }$ 0.2136 0.1925 0.9796 0.0285 0.0011 2 0.2038 0.1847 0.9827 0.0206 8.96E-4 3 0.1734 0.1513 0.9965 0.0025 2.29E-4 4 0.1878 0.1734 0.9892 0.0102 4.87E-4 5 0.2124 0.1806 0.9843 0.0188 7.85E-4 6 0.191 0.1564 0.9896 0.0081 3.86E-4 7 0.2068 0.1886 0.9754 0.0292 0.0012 1阶模型 VR0 k 1 $\rm {V_R} = {V_{R0} }exp\left( { - kt} \right)$ 0.9399 0.0829 0.8383 0.2255 0.0084 2 0.9461 0.0999 0.8733 0.1509 0.0066 3 0.7968 0.1246 0.8341 0.1153 0.0061 4 0.8703 0.1086 0.835 0.1593 0.0072 5 0.9302 0.0923 0.8687 0.1576 0.0066 6 0.8074 0.1014 0.9009 0.0773 0.0037 7 0.9228 0.0908 0.8594 0.1666 0.0069 Weibull分布函数 α β 1 $\rm { {\text{V} }_{\text{R} } } = exp[ - {\left(\dfrac{t}{\alpha }\right)^\beta }]$ 10.9188 0.7816 0.8826 0.1616 0.0062 2 9.2295 0.7884 0.902 0.1168 0.0051 3 5.6397 0.6511 0.907 0.0646 0.0034 4 7.462 0.7058 0.8894 0.1068 0.0049 5 9.7687 0.7697 0.9024 0.1172 0.0049 6 7.1387 0.6707 0.9512 0.0381 0.0018 7 9.8225 0.7597 0.8961 0.1231 0.0051 当决定系数R2最高，残差平方和RSS和卡方χ2最低时，试验值与计算值之间的差异度最小，模型可以最准确地描述干燥过程[26-27]。由表2的拟合结果计算可知，Quadratic模型的R2的取值范围为0.9769~0.9965；RSS的取值范围为0.0024~0.0274；χ2的取值范围为1.34E-4~0.0011。对比五种体积收缩模型发现Quadratic模型的R2值最大，RSS和χ2最小，故Quadratic模型可以更好地展示火龙果热泵干燥过程中体积收缩规律，是描述火龙果热泵干燥的最佳模型。
2.3.2 模型中参数的确定
Quadratic模型中的参数a、b、c与试验条件，即干燥温度（T，℃）、切片厚度（L，mm）和相对湿度（RH，%）有关，不同的试验条件对应不同的a、b、c值。因此，可将参数a、b、c定义为这些变量的一次函数[28]，即
$$\rm a = {\alpha _A}+{\beta _A}T+{\gamma _A}L+{\chi _A}RH $$ (11) $$\rm b = {\alpha _B}+{\beta _B}T+{\gamma _B}L+{\chi _B}RH $$ (12) $$\rm c = {\alpha _C}+{\beta _C}T+{\gamma _C}L+{\chi _C}RH $$ (13) 式中：α、β、γ和χ为待定系数。
利用Quadratic模型的拟合结果对参数a、b、c进行线性拟合，得到待定系数α、β、γ和χ的值，其结果如表3所示。
表 3 参数a、b、c的待定系数值Table 3. Undetermined coefficients of parameters a, b and c待定系数 α β γ χ a 0.2763 −0.0016 0.0022 −0.0007 b 0.2279 −0.0034 0.0194 0.000515 c 0.0609 -0.00038 0.0107 0.00029 将参数a、b、c的各个系数代入公式（11）、（12）和（13）中得：
$$\rm a = 0.2763 - 0.0016T+0.0022L - 0.0007 RH $$ (14) $$\rm b = 0.2279 - 0.0034T+0.0194L+0.000515RH $$ (15) $$\rm c = 0.609+0.00038T+0.0107L+0.00029RH $$ (16) 将拟合所得的模型参数a、b、c代入Quadratic模型，得到火龙果片热泵干燥数学模型为：
$$ \begin{split} \rm {V_R} =& \rm (0.2763 - 0.0016T+0.0022L - 0.0007RH) +(0.2279- \\ & \rm 0.0034T+0.0194L+0.000515 RH)MR +(0.609+ \\ & \rm 0.00038 T+0.0107L+0.00029)M{R^2} \\ \end{split} $$ (17) 2.3.3 模型的验证
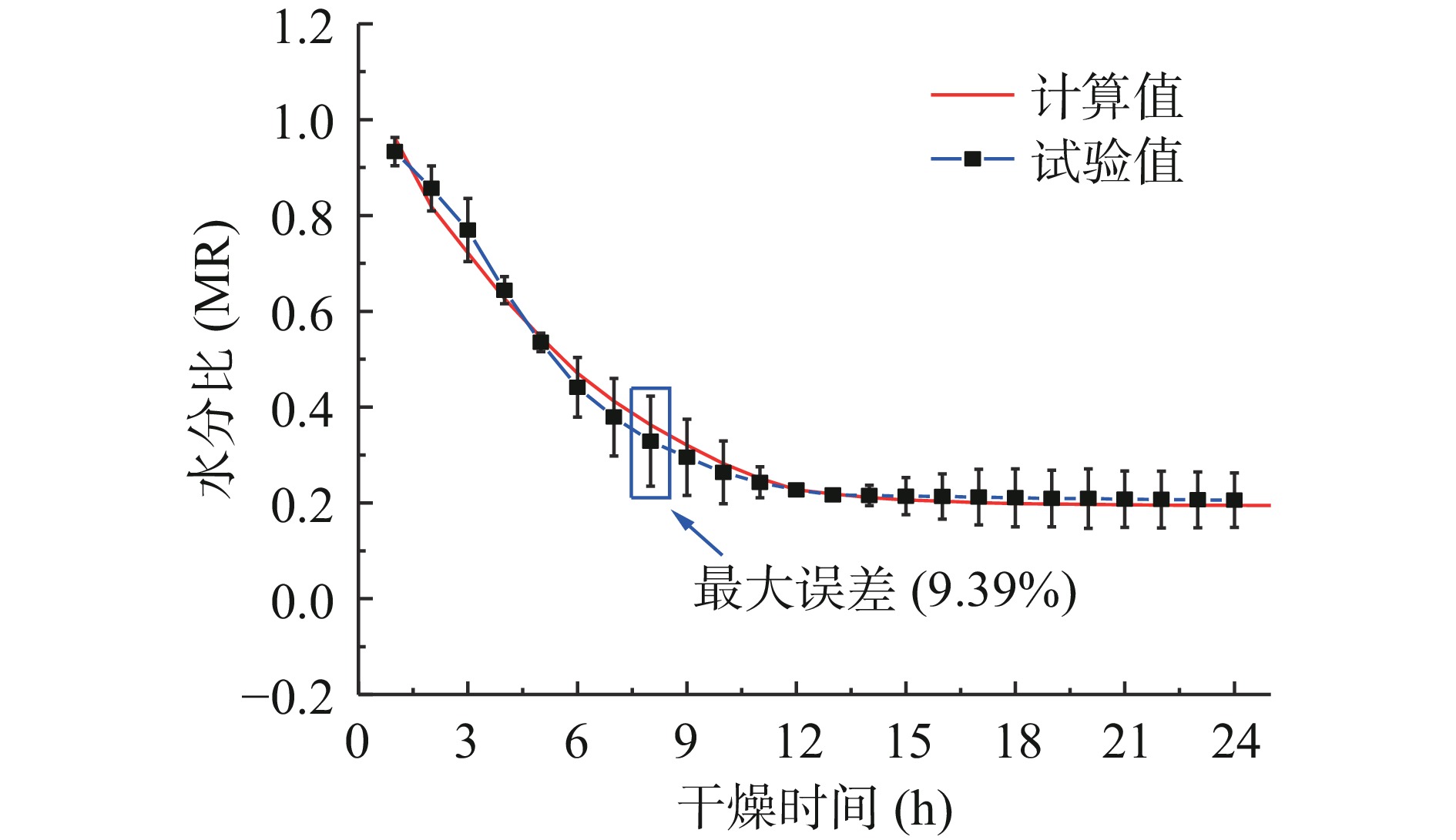
为检测拟合模型的准确性，在干燥温度60 ℃、切片厚度9 mm、相对湿度20%的干燥条件下进行验证试验，试验值与拟合值对比结果如图3所示。拟合值相对于试验值平均误差为5.01%，拟合效果良好。说明Quadratic模型可以较好地预测火龙果热泵干燥过程中的体积收缩规律。
2.4 火龙果的收缩活化能
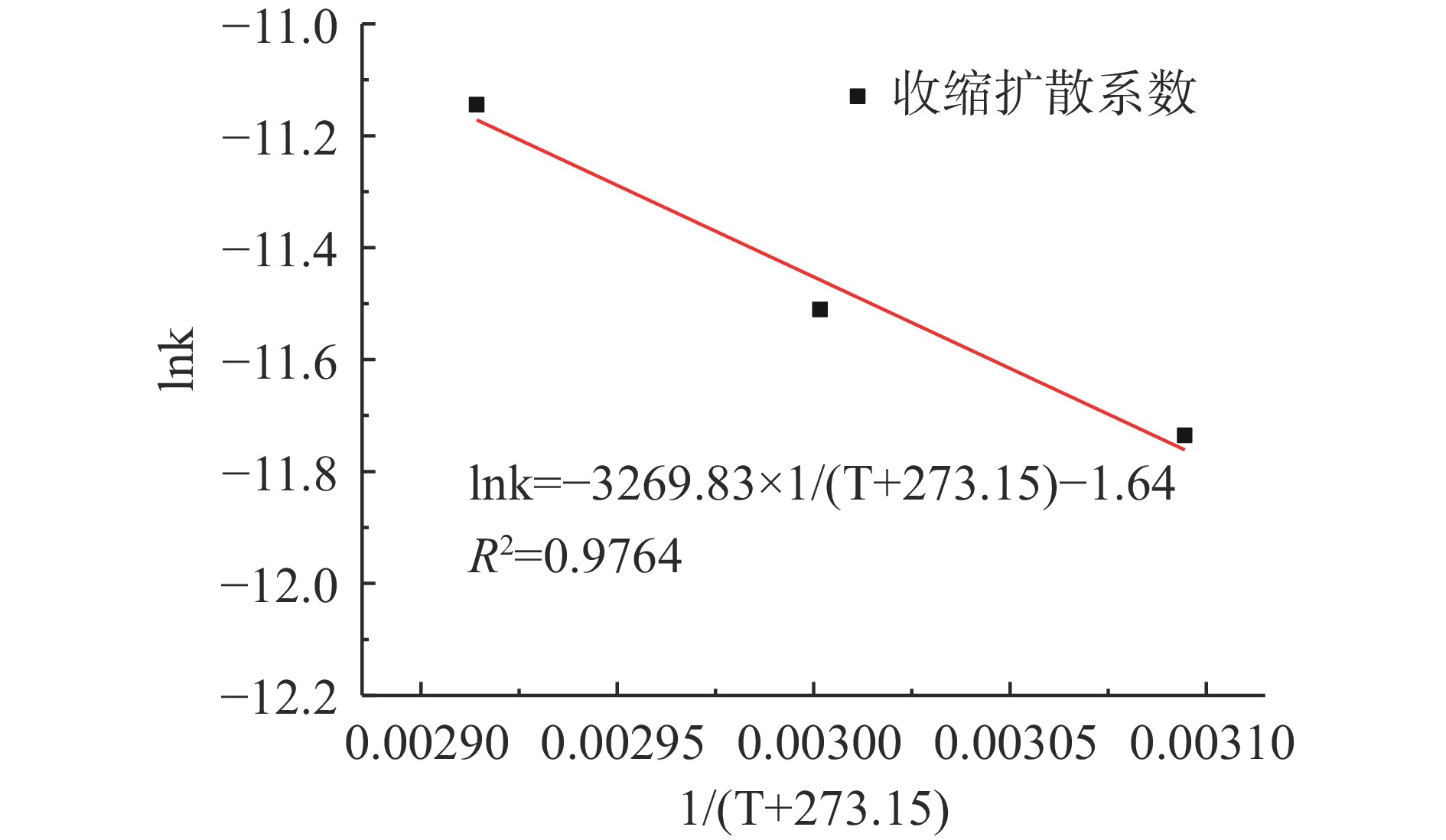
收缩活化能表示在干燥过程中物料体积收缩所需的能量，其大小表示收缩的难易程度[29]。根据阿累尼乌斯方程，可知lnk与1/（T+273.15）呈线性关系，且斜率为
$- \dfrac{{{E_0}}}{R}$ [30]。由图4可知，在切片厚度为9 mm，相对湿度为20%时，lnk与1/（T+273.15）的倒数对应的斜率为−3269.83，计算可以得出，火龙果的收缩活化能为27.185 kJ/mol。收缩活化能仅与温度有关，在生产过程中应根据所需干制品体积来调整干燥温度，合理控制火龙果片的体积收缩，从而提高干制品品质。
![]()
图 4 不同干燥温度下火龙果的收缩活化能Figure 4. Shrinkage activation energy of pitaya at different drying temperatures3. 结论
本文的主要研究是利用体积收缩模型分析火龙果在热泵干燥过程中的收缩动力学。结果表明，提高干燥温度，降低切片厚度和相对湿度可以有效缩短干燥时间，其中干燥温度影响最明显；较高的干燥温度，较低的切片厚度和相对湿度可以提高火龙果片的体积比。对比分析五种体积收缩模型，发现Quadratic模型最适合描述火龙果热泵干燥中的体积收缩过程。根据本文的实验条件，通过收缩动力学模型确定了不同干燥条件下体积比的变化规律，平均误差为5.01%。通过Arrhenius方程计算出火龙果的收缩活化能为27.185 kJ/mol。分析上述结论可知，根据所需干制品的体积来科学控制热泵干燥工艺参数，可以在得到更优品质干制品的同时提高干燥速率，降低干燥能耗，为火龙果热泵干燥的能耗分析和品质改进提供了理论参考。
-
![]()
图 1 不同干燥条件下火龙果水分比和干燥速率曲线
Figure 1. Moisture ratio and drying rate curve of pitaya at different drying conditions
![]()
图 2 不同干燥条件下火龙果体积比曲线
Figure 2. Volume ratio curve of pitaya under different drying conditions
![]()
图 4 不同干燥温度下火龙果的收缩活化能
Figure 4. Shrinkage activation energy of pitaya at different drying temperatures
表 1 体积收缩模型
Table 1 Volume shrinkage model
模型序号 模型名称 模型方程 1 Exponential VR=aexp(bMR) 2 Quadratic VR=a+bMR +cMR2 3 Hatamipour VR=a+bMR 4 1阶 VR=VR0exp(−kt) 5 Weibull分布函数 VR=exp[−(tα)β] 
下载: 导出CSV
表 2 火龙果片热泵干燥过程体积收缩模型的拟合结果
Table 2 Fitting results of shrinkage models of pitaya chips during heat pump drying process
序号 模型表达式 模型参数 R2 RSS χ2 Exponential模型 a b 1 VR=aexp(bMR) 0.2538 0.3488 0.9099 0.1256 0.0047 2 0.2447 0.3416 0.9036 0.1148 0.005 3 0.1993 0.3443 0.9535 0.0323 0.0017 4 0.223 0.349 0.9211 0.075 0.0036 5 0.2515 0.3306 0.9044 0.1148 0.0048 6 0.2217 0.3306 0.92 0.0624 0.003 7 0.2463 0.3533 0.9066 0.1121 0.0047 Quadratic模型 a b c 1 VR=a+bMR +cMR2 0.2081 0.2188 −0.0077 0.9816 0.0256 9.86E-4 2 0.1961 0.2214 −0.0104 0.9871 0.0154 6.99E-4 3 0.1727 0.1549 −9.7E-4 0.9965 0.0024 1.34E-4 4 0.1832 0.197 −0.0067 0.9912 0.0084 4.18E-4 5 0.2038 0.2217 −0.0116 0.9902 0.0118 5.14E-4 6 0.1832 0.195 −0.0108 0.996 0.0031 1.55E-4 7 0.202 0.2099 −0.0063 0.9769 0.0274 0.0011 Hatamipour模型 a b 1 VR=a+bMR 0.2136 0.1925 0.9796 0.0285 0.0011 2 0.2038 0.1847 0.9827 0.0206 8.96E-4 3 0.1734 0.1513 0.9965 0.0025 2.29E-4 4 0.1878 0.1734 0.9892 0.0102 4.87E-4 5 0.2124 0.1806 0.9843 0.0188 7.85E-4 6 0.191 0.1564 0.9896 0.0081 3.86E-4 7 0.2068 0.1886 0.9754 0.0292 0.0012 1阶模型 VR0 k 1 VR=VR0exp(−kt) 0.9399 0.0829 0.8383 0.2255 0.0084 2 0.9461 0.0999 0.8733 0.1509 0.0066 3 0.7968 0.1246 0.8341 0.1153 0.0061 4 0.8703 0.1086 0.835 0.1593 0.0072 5 0.9302 0.0923 0.8687 0.1576 0.0066 6 0.8074 0.1014 0.9009 0.0773 0.0037 7 0.9228 0.0908 0.8594 0.1666 0.0069 Weibull分布函数 α β 1 VR=exp[−(tα)β] 10.9188 0.7816 0.8826 0.1616 0.0062 2 9.2295 0.7884 0.902 0.1168 0.0051 3 5.6397 0.6511 0.907 0.0646 0.0034 4 7.462 0.7058 0.8894 0.1068 0.0049 5 9.7687 0.7697 0.9024 0.1172 0.0049 6 7.1387 0.6707 0.9512 0.0381 0.0018 7 9.8225 0.7597 0.8961 0.1231 0.0051
下载: 导出CSV
表 3 参数a、b、c的待定系数值
Table 3 Undetermined coefficients of parameters a, b and c
待定系数 α β γ χ a 0.2763 −0.0016 0.0022 −0.0007 b 0.2279 −0.0034 0.0194 0.000515 c 0.0609 -0.00038 0.0107 0.00029
下载: 导出CSV
-
[1] 王旭旭, 马领领, 马卓云, 等. 火龙果的功能及其作用机制研究进展[J]. 食品工业科技,2019,40(21):352−360. [WANG X X, MA L L, MA Z Y, et al. Research progress on the function of pitaya and its mechanism[J]. Science and Technology of Food Industry,2019,40(21):352−360. [2] DONG R, LIU S, XIE J, et al. The recovery, catabolism and potential bioactivity of polyphenols from pitaya subjected to in vitro simulated digestion and colonic fermentation[J]. Food Research International,2021,143:110263. doi: 10.1016/j.foodres.2021.110263
[3] 董文丽, 巩雪, 侯理达, 等. 壳聚糖/柠檬酸复合涂膜对火龙果的保鲜效果[J]. 包装工程,2021,42(9):72−78. [DONG W L, GONG X, HOU L D, et al. Effects of chitosan and citric acid composite film on preservation of pitaya[J]. Packaging Engineering,2021,42(9):72−78. [4] 马国军, 刘英, 李武强, 等. 基于响应面法优化火龙果切片远红外干燥工艺[J]. 中国农机化学报,2019,40(12):106−112. [MA G J, LIU Y, LI W Q, et al. Optimization of pitaya slice deep infrared drying process based on response surface methodology[J]. Journal of Chinese Agricultural Mechanization,2019,40(12):106−112. [5] HOU H, CHEN Q, BI J F, et al. Understanding appearance quality improvement of jujube slices during heat pump drying via water state and glass transition[J]. Journal of Food Engineering,2019,272(3):109874.
[6] MAYOR L, SERENO A M. Modelling shrinkage during convective drying of food materials: A review[J]. Journal of Food Engineering,2004,61(3):373−386. doi: 10.1016/S0260-8774(03)00144-4
[7] 刘鹤, 焦俊华, 田友, 等. 马铃薯片热风干燥特性及收缩动力学模型[J]. 食品工业科技,2022,43(11):58−64. [LIU H, JIAO J H, TIAN Y, et al. Study on hot-air drying characteristics and shrinkage dynamics model of potato chips[J]. Science and Technology of Food Industry,2022,43(11):58−64. [8] 陈良元, 韩李锋, 李旭, 等. 茄子片热风干燥收缩特性及其修正的湿分扩散动力学模型[J]. 农业工程学报,2016,32(15):275−281. [[ CHEN L Y, HAN L F, LI X, et al. Structural shrinkage characteristics and modified moisture diffusion kinetics model of sliced eggplant dried by hot air[J]. Transactions of the Chinese Society of Agricultural Engineering,2016,32(15):275−281. doi: 10.11975/j.issn.1002-6819.2016.15.038 [9] DHALSAMANT K, TRIPATHY P P, SHRIVASTAVA S L. Heat transfer analysis during mixed-mode solar drying of potato cylinders incorporating shrinkage: Numerical simulation and experimental validation[J]. Food and Bioproducts Processing,2018,109:107−121. doi: 10.1016/j.fbp.2018.03.005
[10] BURMESTER K, EGGERS R. Heat and mass transfer during the coffee drying process[J]. Journal of Food Engineering,2010,99(4):430−436. doi: 10.1016/j.jfoodeng.2009.12.021
[11] SANDOVAL T S, SOLEDAD T A, HERNANDEZ B E. Dimensionless modeling for convective drying of tuberous crop (Solanum tuberosum) by considering shrinkage[J]. Journal of Food Engineering,2017,214:147−157. doi: 10.1016/j.jfoodeng.2017.06.014
[12] 陈衍男, 王晓, 穆岩, 等. 天麻蒸制后红外干燥特性及失水动力学研究[J]. 食品工业科技,2018,39(22):30−34,40. [CHEN Y N, WANG X, MU Y, et al. Drying characteristics and kinetics research of Gastrodia elata blume under infrared blast drying after steaming[J]. Science and Technology of Food Industry,2018,39(22):30−34,40. [13] ELMIZADEH A, SHAHEDI M, HAMDAMI N. Comparison of electrohydrodynamic and hot-air drying of the quince slices[J]. Innovative Food Science & Emerging Technologies,2017,43:130−135.
[14] OJEDIRAN J O, OKONKWO C E, ADEYI A J, et al. Drying characteristics of yam slices (Dioscorea rotundata) in a convective hot air dryer: Application of ANFIS in the prediction of drying kinetics[J]. Heliyon,2020,6(3):e03555. doi: 10.1016/j.heliyon.2020.e03555
[15] 沈素晴, 徐亚元, 李大婧, 等. 青香蕉微波干燥特性及动力学模型研究[J]. 食品工业科技,2022,43(14):110−117. [SHEN S Q, XU Y Y, LI D J, et al. Research on microwave drying characteristics and kinetic model of green bananas[J]. Science and Technology of Food Industry,2022,43(14):110−117. [16] LI X, LIU Y, GAO Z, et al. Computer vision online measurement of shiitake mushroom (Lentinus edodes) surface wrinkling and shrinkage during hot air drying with humidity control[J]. Journal of Food Engineering,2021,292:110253. doi: 10.1016/j.jfoodeng.2020.110253
[17] 白竣文, 田潇瑜, 刘宇婧, 等. 大野芋薄层干燥特性及收缩动力学模型研究[J]. 中国食品学报,2018,18(4):124−131. [BAI J W, TIAN X Y, LIU Y J, et al. Studies on drying characteristics and shrinkage kinetics modelling of Colocasia gigantea slices during thin layer drying[J]. Journal of Chinese Institute of Food Science and Technology,2018,18(4):124−131. [18] 徐庚, 马月虹, 王庆惠, 等. 芜菁干燥特性及收缩动力学模型研究[J]. 农机化研究,2021,43(10):142−149. [XU G, MA Y H, WANG Q H, et al. Study on physical and dynamical character of the hydroponic butter lettuce[J]. Journal of Agricultural Mechanization Research,2021,43(10):142−149. [19] SEERANGURAYAR T, AL-ISMAILI A M, JEEWANTHA L H J, et al. Experimental investigation of shrinkage and microstructural properties of date fruits at three solar drying methods[J]. Solar Energy,2019,180(Mar.):445−455.
[20] NANVAKENARIA S, MOVAGHARNEJAD K, LATIFI A. Modelling and experimental analysis of rice drying in new fluidized bed assisted hybrid infrared-microwave dryer[J]. Food Research International,2022,159:111617. doi: 10.1016/j.foodres.2022.111617
[21] DEHGHANNYA J, KADKHODAEI S, HESHMATI M K, et al. Ultrasound-assisted intensification of a hybrid intermittent microwave hot-air drying process of potato: Quality aspects and energy consumption[J]. Ultrasonics,2019,96:104−122. doi: 10.1016/j.ultras.2019.02.005
[22] RAMIREZ C, ASTORGA V, NUNEZ H, et al. Anomalous diffusion based on fractional calculus approach applied to drying analysis of apple slices: The effects of relative humidity and temperature[J]. Journal of Food Process Engineering,2017,40(5):e12549. doi: 10.1111/jfpe.12549
[23] 王迪芬, 苑亚, 魏娟, 等. 苹果热风干燥工艺优化和特性分析[J]. 食品工业科技,2021,42(1):144−148,155. [WANG D F, YUAN Y, WEI J, et al. Optimization and characteristic analysis of apple hot-air drying process[J]. Science and Technology of Food Industry,2021,42(1):144−148,155. [24] DHURVE P, ARORA V K, YADAV D K, et al. Drying kinetics, mass transfer parameters, and specific energy consumption analysis of watermelon seeds dried using the convective dryer[J]. Mater Today Proceedings,2022,59:926−932. doi: 10.1016/j.matpr.2022.02.008
[25] MALAKAR S, ARORA V K. Mathematical modeling of drying kinetics of garlic clove in forced convection evacuated tube solar dryer[J]. Advances in Fluid and Thermal Engineering,2021:813−820.
[26] 兰大为, 赵芳, 王玉清, 等. 热风干燥条件对干燥特性影响及数学模型研究[J]. 内蒙古石油化工,2022,48(4):54−58. [LAN D W, ZHAO F, WANG Y Q, et al. Effect of hot-air drying conditions on drying characteristics and mathematical model[J]. Inner Mongolia Petrochemical Industry,2022,48(4):54−58. [27] MUTHUKUMAR P, LAKSHMI D, KOCH P, et al. Effect of drying air temperature on the drying characteristics and quality aspects of black ginger[J]. Journal of Stored Products Research,2022,97:101966. doi: 10.1016/j.jspr.2022.101966
[28] 王航. 香蕉片高压电场-热泵联合干燥特性研究[D]. 郑州: 中原工学院, 2021. WANG H. Study on drying characteristics of banana slices with high pressure electric field and heat pump[D]. Zhengzhou: Zhongyuan University of Technology, 2021.
[29] 黄光群, 余浩, 方晨, 等. 奶牛粪固形物热风干燥特性及工艺参数优化[J]. 农业工程学报,2021,37(23):186−193. [HUANG G Q, YU H, FANG C, et al. Hot-air drying characteristics and optimization of the process parameters for the solid fraction of dairy manure[J]. Transactions of the Chinese Society of Agricultural Engineering,2021,37(23):186−193. [30] 汤尚文, 马雪伟, 于博, 等. 马铃薯红外干燥特性研究[J]. 保鲜与加工,2018,18(1):76−81,89. [TANG S W, MA X W, YU B, et al. Infrared radiation drying characteristics of potato[J]. Storage and Process,2018,18(1):76−81,89. doi: 10.3969/j.issn.1009-6221.2018.01.013
下载:
计量
- 文章访问数: 163
- HTML全文浏览量: 31
- PDF下载量: 12
